
This slide rule has a logarithmic scale. Logarithms were discovered by John Napier (1550–1617). On a log scale, the spacing between each multiple of 10 is equal, while the spacing between individual numbers changes along the rule. So the space from 1 to 10 is the same as the space from 10 to 100, and so on! (This slide rule restarts labels at 1 for each higher multiple of 10 to save space.)


A slide rule is a mechanical analog computer that can be used to do many types of calculations, including multiplying and dividing. The first slide rule using multiple scales was designed by William Oughtred (1575 –1660).
What to do
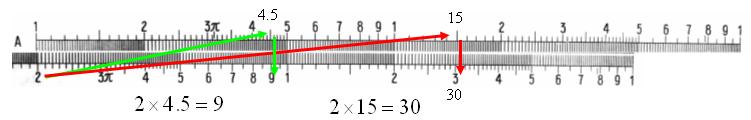
- Move the middle of the slide rule so that the first number 2 on line B lines up with the first number 1 on line A.
- We are set up to multiply 2 by any number on line A.
- We read the solution (the number directly below) off line B.
| WHAT IS 2 x 4.5? | WHAT IS 2 x 15? |
| Answer: | |
 |
|
- Try multiplying by other numbers!
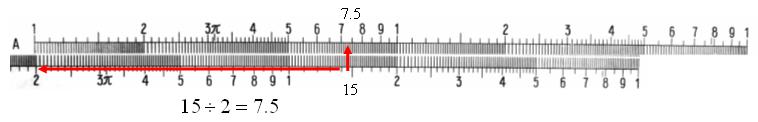
CAN YOU FIGURE OUT HOW TO USE THE SLIDE RULE FOR DIVISION?
For division, we line up the number we want to divide by on line B with the first number 1 on line A. Then we find our dividend on line B and the quotient (solution) will be directly above it on line A.
WHAT IS 15÷2?
Answer:
What is going on?
The lengths on our scale correspond to logarithms of each number. Logarithms have the property that the logarithm of two numbers multiplied together is equal to adding up the logarithm of each number individually. The logarithm of a number divided by another is equal to subtracting the logarithm of each number individually.
Using examples from above:
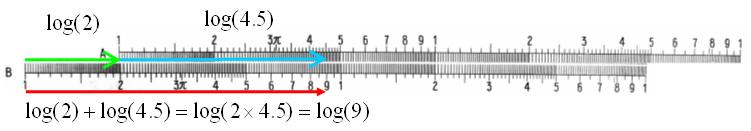
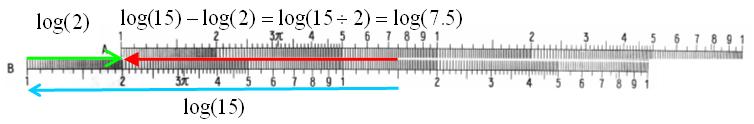
Algebraically: log(x) + log(y) = log(xy) and log(x) – log(y) = log(x/y)