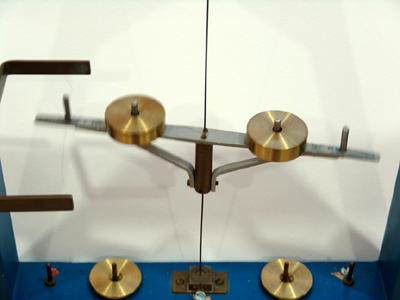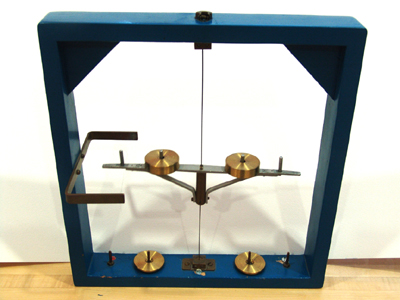
L.R. Ingersoll Physics Museum
UW–⁠Madison Department of Physics

Torsion Pendulum

 

In torsion pendulums (like the one used in some clocks), the oscillation speed depends on the moment of inertia of the pendulum system and the spring constant of the wire, which is a measure of how torque is required to twist the wire through some angle. (See the Angular Momentum Turntable exhibit for more on the moment of inertia.)

Experiment 1: WHAT TO DO:

  1. Place the two large masses on the outer two pins of the torsion arm.
  2. Pull one side of the torsion arm towards you a small distance and then let it go.
  3. Count the time it takes the arm to make ten oscillations.
  4. Replace the large masses with the two small masses in the same spots.
  5. Pull one side of the torsion arm towards you the same small distance as before and let it go.
  6. Count the time it takes the arm to make ten oscillations.

HOW DO THE OSCILLATION TIMES (PERIODS) COMPARE?

Answer: The period of the torsion arm with the larger masses is twice as long as the period of the arm with the smaller masses.

Experiment 2: WHAT TO DO:

  1. Put the small masses on the same outer two pins of the torsion arm.
  2. Pull one side of the torsion arm towards you a small distance and then let it go.
  3. Count the time it takes the arm to make ten oscillations.
  4. Remove the small masses.
  5. Place the two large masses on the INNER two pins of the torsion arm.
  6. Pull one side of the torsion arm towards you the same small distance as before and let it go.
  7. Count the time it takes the arm to make ten oscillations.

HOW DO THE OSCILLATION TIMES (PERIODS) COMPARE?

Answer: The period of the torsion arm with the smaller masses is equal to the period of the torsion arm with the larger masses.

What is going on? The moment of inertia, I, depends on the mass on the pendulum and its distance from the center of rotation of the pendulum. A smaller the moment of inertia increases the oscillation frequency and decreases the period of the pendulum. In Exp.1, the pendulum has a higher I with the larger masses than with the small, so the period is longer than with the small masses. In Exp. 2, the moment of inertia for the small masses at a big distance is equal to the moment of inertia for the big masses at a small distance, so the periods are the same in both cases.

Original 1918 Museum Exhibit

Physics Lecture Demonstration Database